What is ker F?

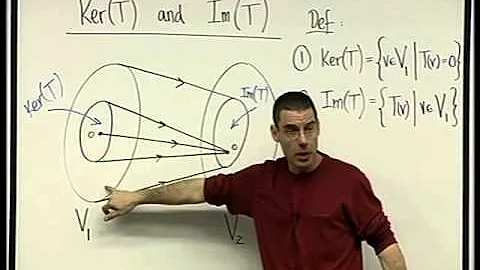
What is ker F?

Kerf = Width Kerf is defined as the width of material that is removed by a cutting process.

What is kerf in math?

The usual definition for the kernel of a group homomorphism f:G→H is that ker(f) consists of all those elements in the domain which are mapped to the identity of the codomain. That is, {x∈G:f(x)=eH}.

What is a kernel of homomorphism?

In algebra, the kernel of a homomorphism (function that preserves the structure) is generally the inverse image of 0 (except for groups whose operation is denoted multiplicatively, where the kernel is the inverse image of 1). An important special case is the kernel of a linear map.

How do you find the kernel of a function?

To find the kernel of a matrix A is the same as to solve the system AX = 0, and one usually does this by putting A in rref. The matrix A and its rref B have exactly the same kernel. In both cases, the kernel is the set of solutions of the corresponding homogeneous linear equations, AX = 0 or BX = 0.

Can the kernel be empty?

The kernel of a linear transformation is never empty, since for any vector spaces $V$ and $W$, and any linear transformation $f:V\to W$, it must be true that $$f(0_V)=0_W$$ and therefore $0_V\in\ker(f)$.

What does it mean for a kernel to be trivial?

Injective ⟹ the kernel is trivial Suppose the homomorphism f:G→H is injective. Then since f is a group homomorphism, the identity element e of G is mapped to the identity element e′ of H. Namely, we have f(e)=e′. If g∈ker(f), then we have f(g)=e′, and thus we have.

What is the trivial kernel?

Injective ⟹ the kernel is trivial Namely, we have f(e)=e′. If g∈ker(f), then we have f(g)=e′, and thus we have. f(g)=f(e). Since f is injective, we must have g=e.

What is an F vector space?

The general definition of a vector space allows scalars to be elements of any fixed field F. The notion is then known as an F-vector space or a vector space over F. A field is, essentially, a set of numbers possessing addition, subtraction, multiplication and division operations.

Is kernel a subgroup?

The kernel of φ, denoted Ker φ, is the inverse image of the identity. Then Ker φ is a subgroup of G. Proof. We have to show that the kernel is non-empty and closed under products and inverses.

What is the kernel of a ring?

Similarly, in ring theory, the kernel of a homomorphism is the inverse image of zero; the kernel is a two-sided ideal of the ring, and every two-sided ideal of a ring is the kernel of a ring homomorphism.

What does the term "kerf" mean in woodworking?

• Joinery. This is simply the practice of connecting pieces of wood together through various means. There are many types of joinery that exist.
• Kerf. Another important term that you need to know is kerf. ...
• Abrasive. If you are interested in going further in discovering others,you can check out this glossary. ...

What is kerf in laser cutting?

• The laser burns away a portion of material when it cuts through. This is known as the laser kerf and ranges from 0.08mm – 1mm depending on the material type and other conditional factors. Although above c0.45mm is only experienced when cutting thicker foams.

What is kerf in welding?

• Kerf may refer to: Kerf, the width of a saw cut. Kerf, molten metal and metal oxide blown out when metal is cut by an oxy-gas torch: see Oxy-fuel welding and cutting. Kerf, a poetry collection by Peter Sanger . Kerf dust, a byproduct of sawing silicon ingots into wafers, esp. in production of Photovoltaics .

What is kerf on saw blades?

• Saw blade “kerf” refers to the thickness of the slot which the saw blade will cut. It is often used as well to define the thickness of the blade itself, or at least the widest point on the blade, as this will define the width of cut made.